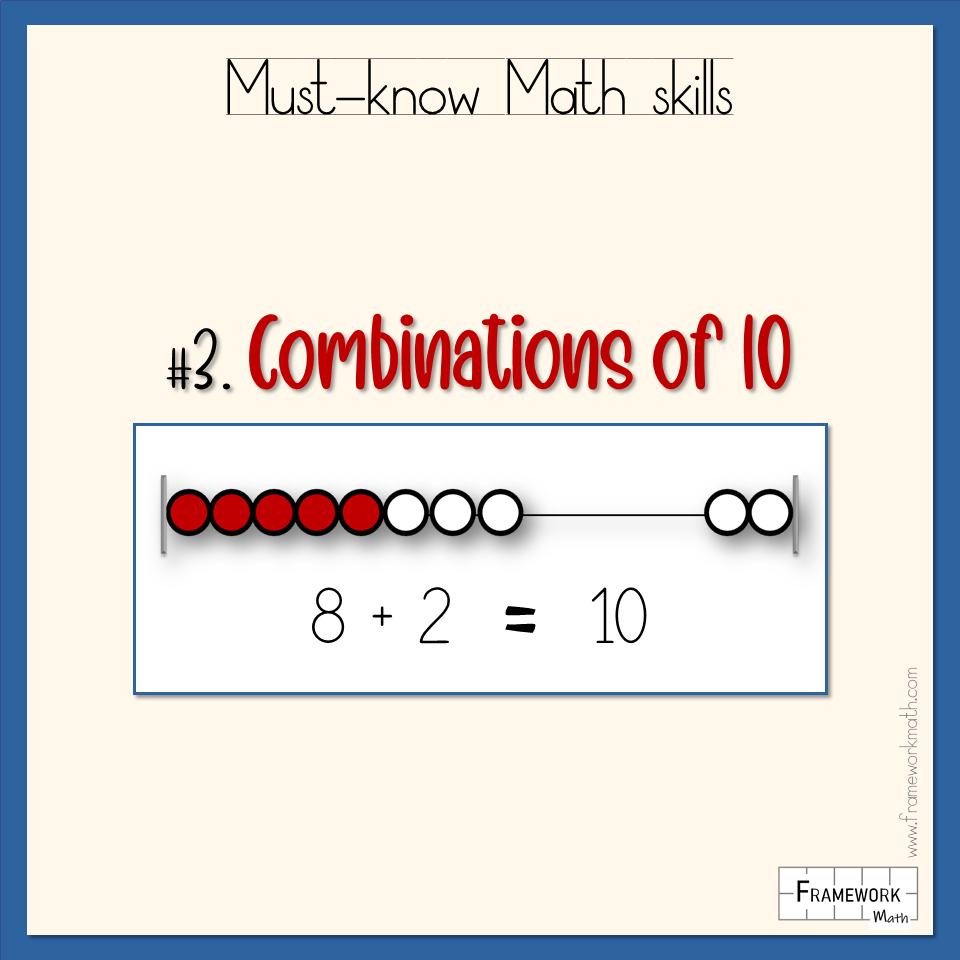
Why are the combinations of 10 key to unlocking number sense?

Part 3 in our #numbersenseseries: Combinations of 10

The combinations of 10 are quite possibly the most useful set of facts to know automatically, and should be constantly reviewed to make sure they are well-known, well-loved, comfortable numbers.

Rather than just focusing on memorizing all the number combinations however, help students build and see the combinations in a ten frame or on a Rekenrek bar, where the groups of 5 are either a different row (on a ten frame) or a different color (on a Rekenrek) so that the brain can rely on instant visual cues without being slowed down by counting.

I like to explain this concept, when it's introduced for the first time, as "the other side of the moon."

When you see a sliver of the moon, do you think the other part is missing? No; we know that the whole moon is there, but we can only see part of it.

Whenever you see a number (single-digit), you also know that the "other part" is there that completes the group of 10. So when you see the number 8, you know that it would take 2 more to complete the 10.

When students know these facts automatically, not based on pure memorization, but based on familiarity with the tactile experience and visual images, they will have a huge advantage when it comes to adding and subtracting numbers.

For a simple game idea, you can have students write several one-digit numbers on the board, or use playing cards and spread them out around the floor, then play "clean it up."

You can also do this with a 10-row Rekenrek by sliding over a random amount of beads on each row to start the game.

Students will collect as many groups of 10's as possible, crossing out the numbers as they get used and keeping track of how many tens they make.

Then when they get down to a set of numbers with no obvious combinations of 10 left, tell them they get to be detectives and look for "hiding 10's" in the remaining numbers.

See if they can recognize that there is a hiding 10 in a set of numbers like 6 and 5, since they can combine the 5's and then 1 will be left over.

Use the Rekenrek or double ten frames to model this if it's still needed.

After playing this game several times, you can write a vertical stack of numbers and try transitioning the same tactile skills to paper. This should help students experience addition in a fun way, where they are always on the hunt for 10's, whether they are obvious combinations or hiding 10's.

Then without even realizing it, they'll have journeyed into double-digit addition with regrouping, just by learning to subitize within 10 and knowing their combinations of 10.

Follow us on Facebook (page or group) or browse the blog posts here for more quick tips on using a Rekenrek to help your learners build a conceptual understanding of Math, rather than simply memorize Math facts and procedures — and feel free to share if you find these helpful!