Those tricky teenager numbers …
Part 4 in our #numbersenseseries: The "10 plus-es"
The "teenager numbers" can be tricky for students who are just beginning to learn their numbers. In English, the names of the numbers from 11 through 20 don't tell us very much about the place value structure of those numbers.
If, for instance, the number 12 were called "ten-two" instead of "twelve," students would be able to understand, from the very beginning of their Math journey, how numbers are built (composed) in groups of 1's and 10's. They would see 12 as a group of 10 beads (if you are using a Rekenrek) and 2 extra beads.
Even our "decade" numbers could be named more accurately; if twenty-one were instead called "two tens one" then students would see the inherent place value within the name of the number itself.
In some languages, numbers are named this way; this is a big advantage to students because it removes the middle step of connecting a seemingly random number name to a mental picture of the way that number is composed.
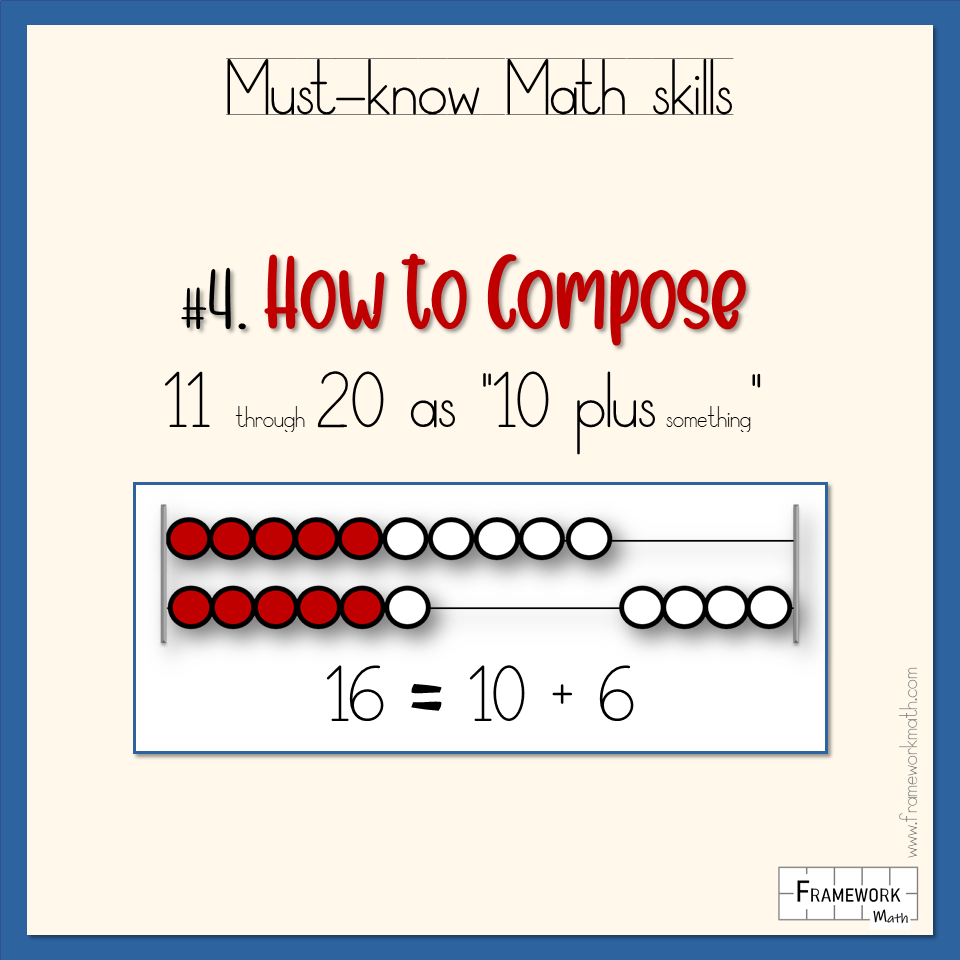
But for those teaching Math in English, we do need to go that extra step to zoom in and really focus on the numbers from 11 - 20, helping students build the numbers in a double ten frame or a 2 bar Rekenrek, count them, and learn to instantly recognize each number as a combination of "10 plus something."
This, as with each of the skills in this series, forms the basis for helping your learners develop a strong number sense - which will help them rely much more on working flexibly with numbers they can visualize in their head rather than blindly following procedures and memorizing isolated facts.
This skill also sets students up well for learning addition strategies, rather than memorizing sums, since they will be able to re-arrange something like 9 + 6 into 10 + 5, which is the same sum, but composed in a more familiar and friendly way.
(Keep an eye out for a 7-part series coming next on addition strategies!)
These basic skills will serve as the key element to becoming fluent in the language of Math and mastering a deep, conceptual understanding of how Math works. :)
Follow us on Facebook (page or group) or browse the blog posts here for more quick tips on using a Rekenrek to help your learners build a conceptual understanding of Math, rather than simply memorize Math facts and procedures — and feel free to share if you find these helpful!
Quick Practice:
Find a Rekenrek.
You can buy one, build one, or use a free online version. See suggestions here.
Help your learner build numbers 11 - 20 on a Rekenrek.
Start with all beads on the right. Slide beads to the left as you use them. Try to use only one push per row to help with subitizing.
Once they are comfortable building numbers, show them a number from 11 through 20 and see if they can instantly recognize how many beads there are without counting.
Ask: Show them any number from 11 - 20 and have them cover up the group of 10 (the top row) and tell you how many are leftover.
Ask: Make the number 17, hide top row of beads, and while the student is looking at the 7 white beads that you haven’t covered ask what the total number of beads is.
Ask: Make the number 16, then hide the beads on the bottom row so only the top row with 10 beads is showing. Tell them you have 16 beads and ask them how many you are hiding.
Ask: Can you picture 19 beads on a Rekenrek in your head? What does it look like?