Place Value: The most important skill of all
Part 5 in our #numbersenseseries: Place Value
Place value is perhaps the most important, foundational skill that students need to thoroughly understand in order to be successful in Math.
It is so much more than just a "side study" on figuring out what digit is in the thousand's place when faced with a 6-figure number.
Place value is the context in which numbers exist and where we operate on them.
Place value sets up an organized, dependable system where groups of 10 live together. Whether that's a group of 10 ones or 10 millions, each value has its own place.
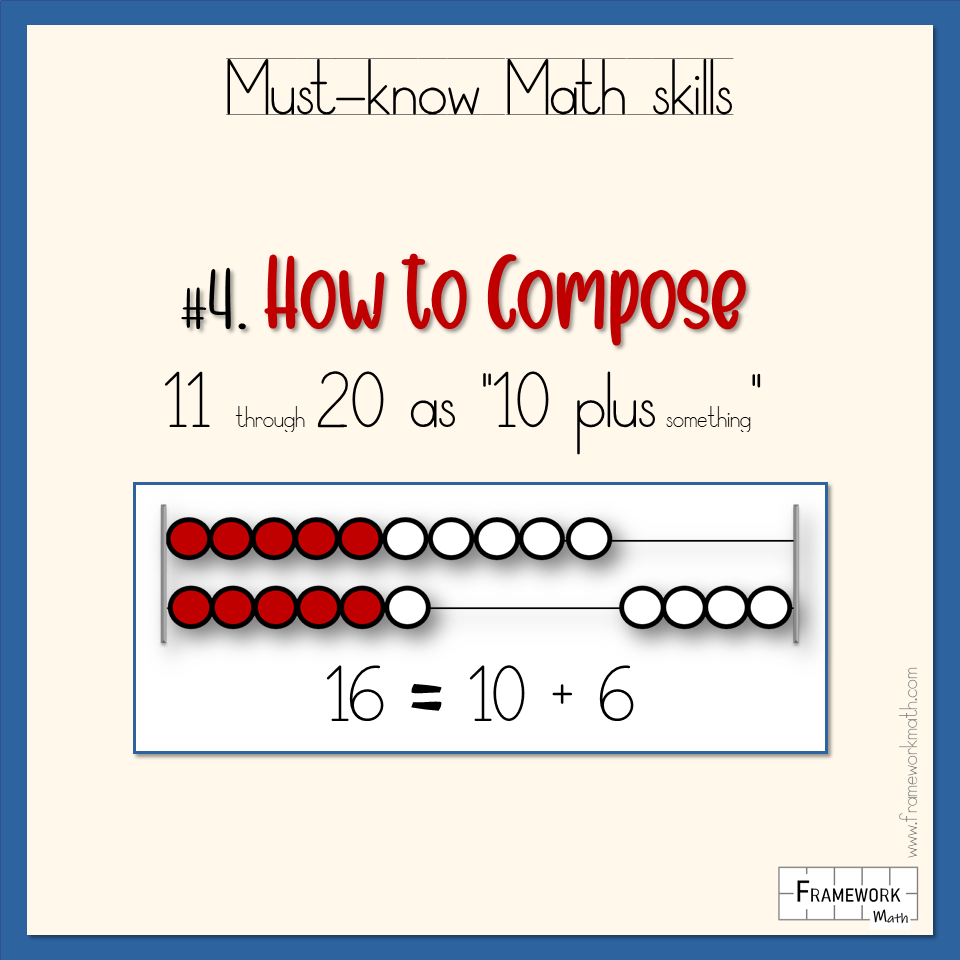
When students construct numbers (like 12 or 12,345) with base 10 blocks or in place value ten frames, they simultaneously construct an intuitive understanding of the "ten-ness" of our number system.
Then the process of deconstructing those numbers becomes a logical extension of these tactile experiences or visual images.
For instance, students will know that 123 is 3 ones, 2 tens (or 20 ones), and 1 hundred (or 10 tens, or 100 ones).
So often students get lost in numberland when they start operating on multi-digit numbers and treating them like collections of single-digits, rather than the whole numbers that they are.
Finding the difference between 104 and 96 for instance, can be as simple as realizing that both numbers are 4 units away from 100, so the difference between them is 8.
Solving this as a "stack-and-subtract" problem with the traditional algorithm, however, can look daunting and throw students off guard with all the regrouping (and that dreaded zero!) that comes into play.
When students understand the value of each "place" in a multi-digit number and can deconstruct it into its separate parts, they will be able to regroup those numbers flexibly - which is at the heart of all 4 operations.
So a number like 58 and 67 can be seen as a group of 10 tens (with one extra ten) and 10 ones (with 5 extra ones) - or 1 hundred, 2 tens, and 5 ones: 125.
The best way to build this magical "sense" about numbers and the value of each place in a number is simply to play around with it.
The more comfortable students become with numbers in their native sets of 10's, the more flexible and confident they'll become with Math.
(Keep an eye out for a 7-part series coming next on addition strategies!)
These basic skills will serve as the key element to becoming fluent in the language of Math and mastering a deep, conceptual understanding of how Math works.
Follow us on Facebook (page or group) or browse the blog posts here for more quick tips on using a Rekenrek to help your learners build a conceptual understanding of Math, rather than simply memorize Math facts and procedures — and feel free to share if you find these helpful!
Quick Practice:
Practice constructing 1-digit numbers in a ten frame, then deconstructing them into 5's and 1's.
Build 2-digit numbers on a Rekenrek, then name the 10's and 1's, and re-name things like 63 as 5 tens and 13 ones.
Build 3-digit numbers and beyond in place value ten frames, then combine it with another 3-digit number, making trades whenever a full group of 10 is reached.